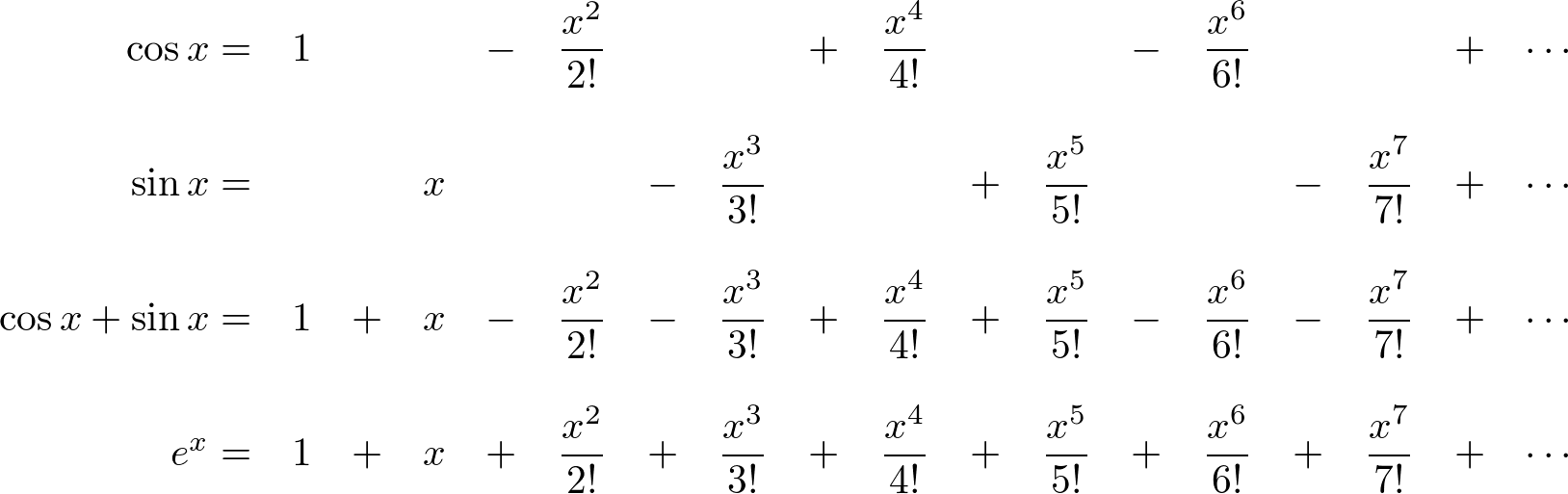Sin X Exponential Form
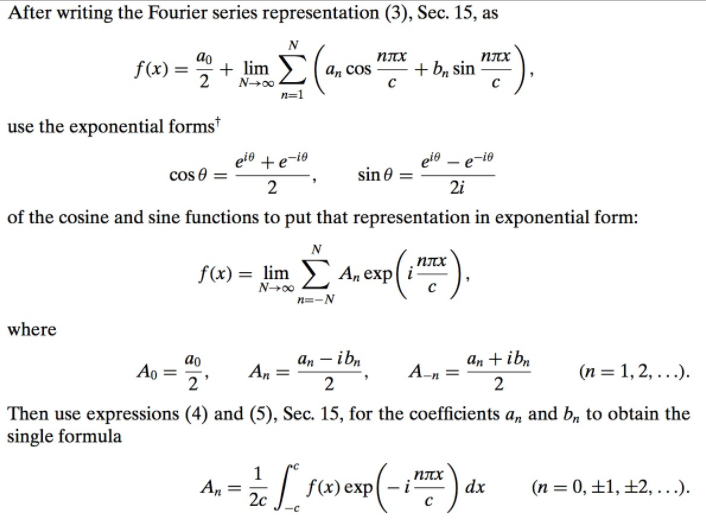
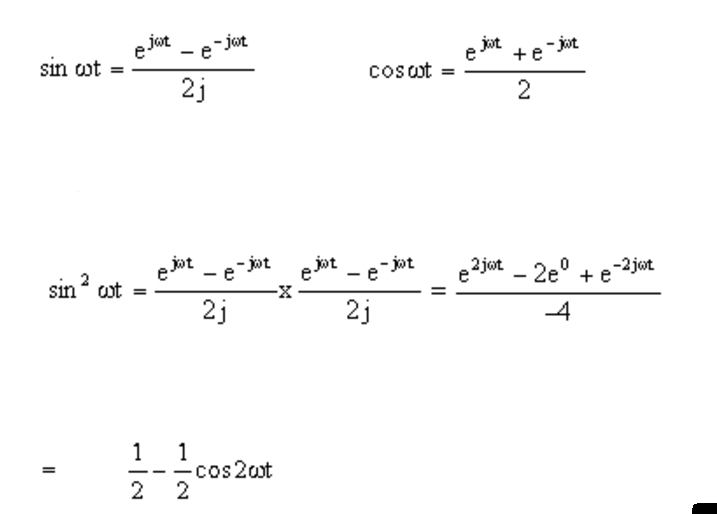
Sin X Exponential Form - [1] the sinc function as audio, at 2000 hz (±1.5. = 1 + x + x 2 2 ! = a + ib one can apply the exponential function to get. Sin t = eit ¡e¡it 2i: Could somebody please explain how this. Web eit = cos t+i sin t; Exp(a + ib) = exp(a) exp(ib) = exp(a)(cos b + i sin b) the trigonmetric addition formulas (equation 1). Sin z = exp(iz) − exp(−iz) 2i sin z = exp ( i z) − exp ( − i z) 2 i. Web in mathematics, physics and engineering, the sinc function, denoted by sinc (x), has two forms, normalized and unnormalized. Exponential form uses the same attributes as polar form, absolute value and angle. Web relations between cosine, sine and exponential functions. For any complex number z z : Exp(a + ib) = exp(a) exp(ib) = exp(a)(cos b + i sin b) the trigonmetric addition formulas (equation 1). F(u) = 1 ju[eju 2 −e−ju 2] f ( u) = 1 j u [ e j u 2 − e − j u 2]. +. R ⋅ e i θ. Web eit = cos t+i sin t; Sin t = eit ¡e¡it 2i: F(u) = 1 ju[eju 2 −e−ju 2] f ( u) = 1 j u [ e j u 2 − e − j u 2]. Cosθ = ejθ +e−jθ 2, sinθ = ejθ −e−jθ 2j 2. Web in order to expand (1 + x)e x as a taylor series in x, we use the known taylor series of function e x: Web in mathematics, physics and engineering, the sinc function, denoted by sinc (x), has two forms, normalized and unnormalized. (45) (46) (47) from these relations and the properties of exponential multiplication you can painlessly prove. It only displays them in a different way that is more compact. = 1 + x + x 2 2 ! The exponential form of a complex number using the polar form, a complex number with. Could somebody please explain how this. E x = ∑ (k=0 to ∞) (x k / k!) = 1 + x + (x 2. F(u) = 1 ju[eju 2 −e−ju 2] f ( u) = 1 j u [ e j u 2 − e − j u 2]. Cos t = eit +e¡it 2; Exponential form uses the same attributes as polar form, absolute value and angle. R ⋅ e i θ. Z denotes the exponential function. E¡it = cos t¡i sin t (4) so adding (and dividing by 2) or subtracting (and dividing by 2 i) gives: Sin z = exp(iz) − exp(−iz) 2i sin z = exp ( i z) − exp ( − i z) 2 i. (45) (46) (47) from these relations and the properties of exponential multiplication you can painlessly prove all.. + x 3 3 ! Web $$ e^{ix} = \cos(x) + i \space \sin(x) $$ so: [1] the sinc function as audio, at 2000 hz (±1.5. Z denotes the exponential function. It only displays them in a different way that is more compact. Web $$ e^{ix} = \cos(x) + i \space \sin(x) $$ so: Exp(a + ib) = exp(a) exp(ib) = exp(a)(cos b + i sin b) the trigonmetric addition formulas (equation 1). For any complex number z z : Could somebody please explain how this. = a + ib one can apply the exponential function to get. E x = ∑ (k=0 to ∞) (x k / k!) = 1 + x + (x 2 / 2!) + (x 3 / 3!) +. + x 4 4 ! Cosθ = ejθ +e−jθ 2, sinθ = ejθ −e−jθ 2j 2. Sin z = exp(iz) − exp(−iz) 2i sin z = exp ( i z) − exp ( −. Web to solve an exponential equation start by isolating the exponential expression on one side of the equation. Web in mathematics, physics and engineering, the sinc function, denoted by sinc (x), has two forms, normalized and unnormalized. Z denotes the exponential function. It only displays them in a different way that is more compact. Could somebody please explain how this. Sin z = exp(iz) − exp(−iz) 2i sin z = exp ( i z) − exp ( − i z) 2 i. Exponential form uses the same attributes as polar form, absolute value and angle. + x 3 3 ! The exponential form of a complex number using the polar form, a complex number with. Web for any complex number. Cosθ = ejθ +e−jθ 2, sinθ = ejθ −e−jθ 2j 2. For any complex number z z : E x = ∑ (k=0 to ∞) (x k / k!) = 1 + x + (x 2 / 2!) + (x 3 / 3!) +. Z denotes the exponential function. (45) (46) (47) from these relations and the properties of exponential multiplication you can painlessly prove all. Web $$ e^{ix} = \cos(x) + i \space \sin(x) $$ so: Cos t = eit +e¡it 2; + x 4 4 ! Web exponentials the exponential of a real number x, written e x or exp(x), is defined by an infinite series,. It only displays them in a different way that is more compact. = a + ib one can apply the exponential function to get. Web eit = cos t+i sin t; Web in mathematics, physics and engineering, the sinc function, denoted by sinc (x), has two forms, normalized and unnormalized. Then, take the logarithm of both sides of the equation to convert the. R ⋅ e i θ.Example 11 Simplify and write the answer in exponential form
Example 5 Express tan1 cosx/(1 sinx) Chapter 2 Inverse
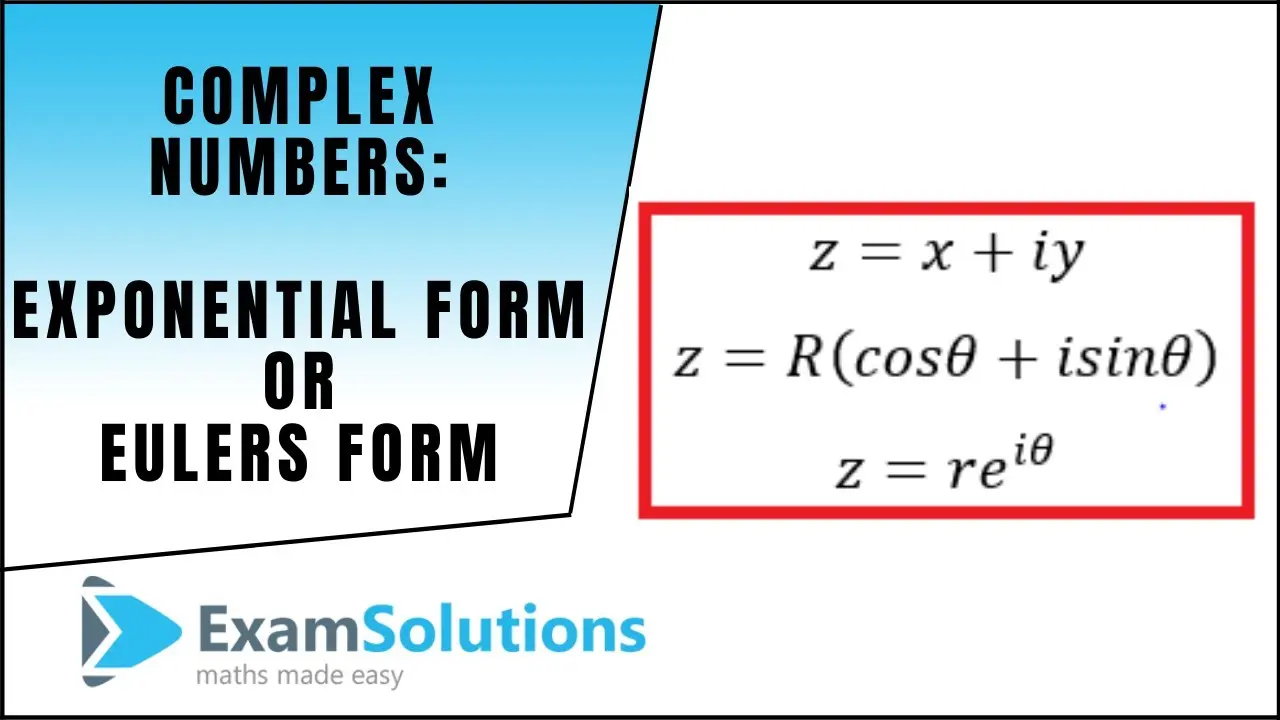
Euler's Equation
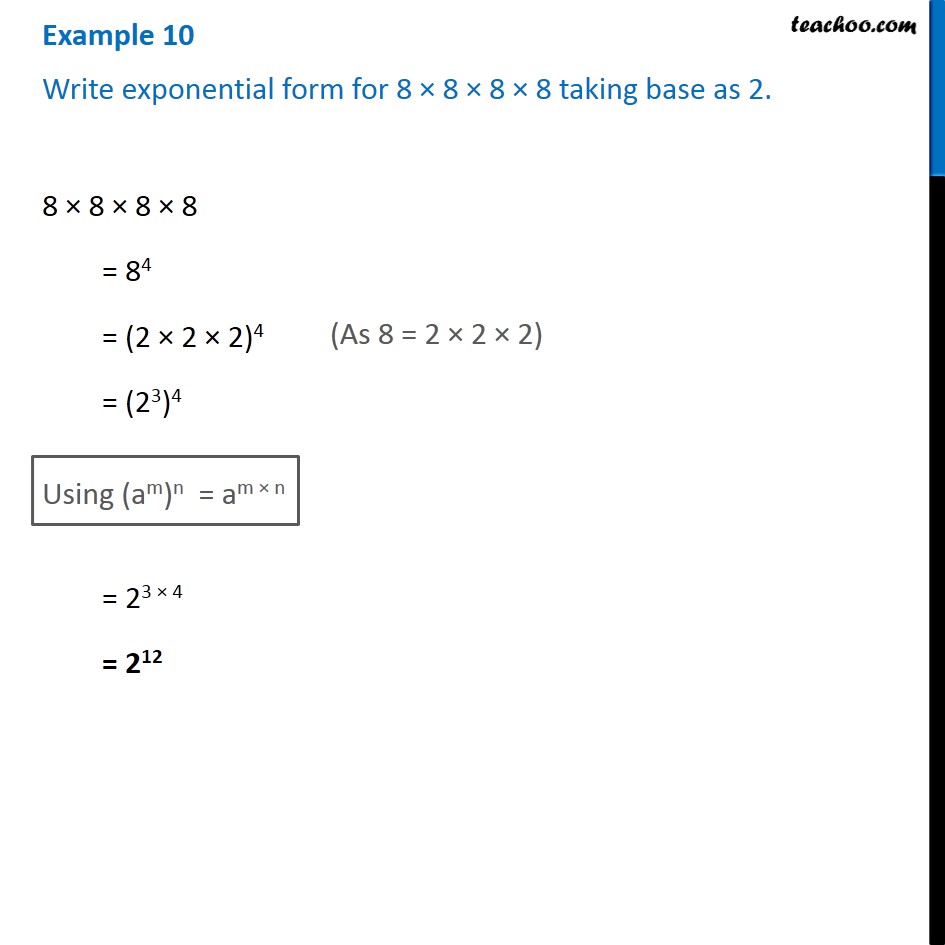
Example 10 Write exponential form for 8 x 8 x 8 x 8 taking base as 2
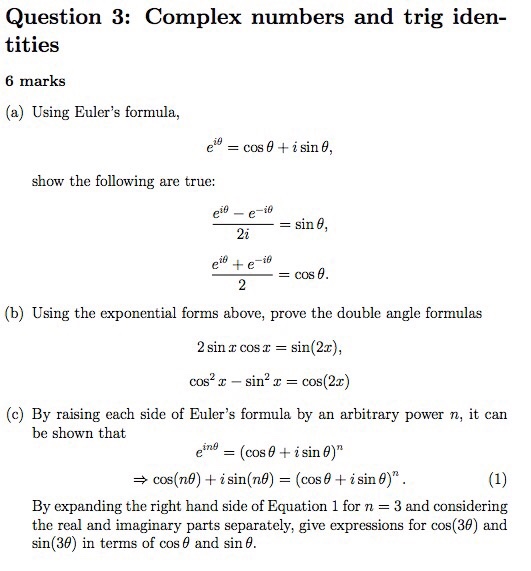
Solved Question 3 Complex numbers and trig iden tities 6
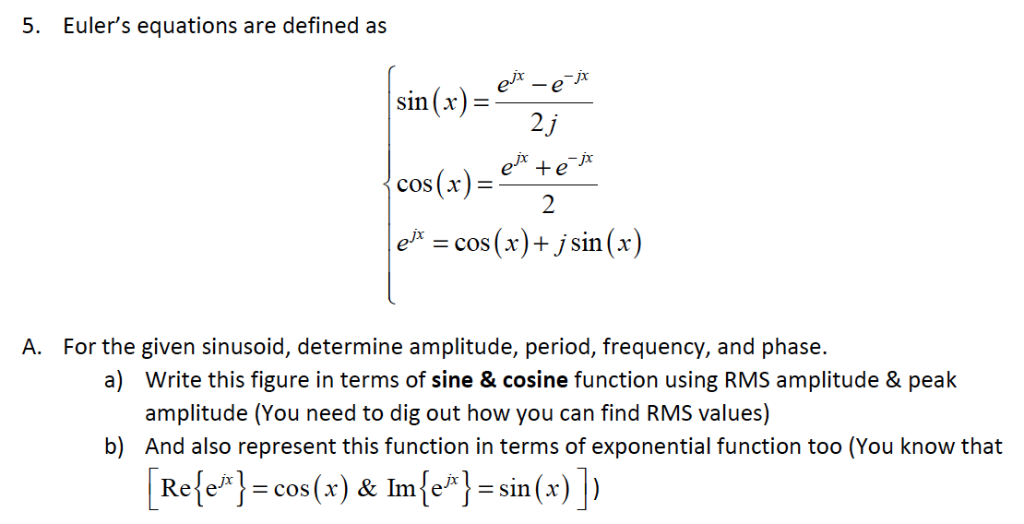
Solved 5. Euler's equations are defined as sin (x) cos(x) e"
Complex number notation forms trigonometric, exponential Healthy
Complex Numbers 4/4 Cos and Sine to Complex Exponential YouTube
Other Math Archive January 29, 2018
Basics of QPSK modulation and display of QPSK signals EE World Online
Related Post: