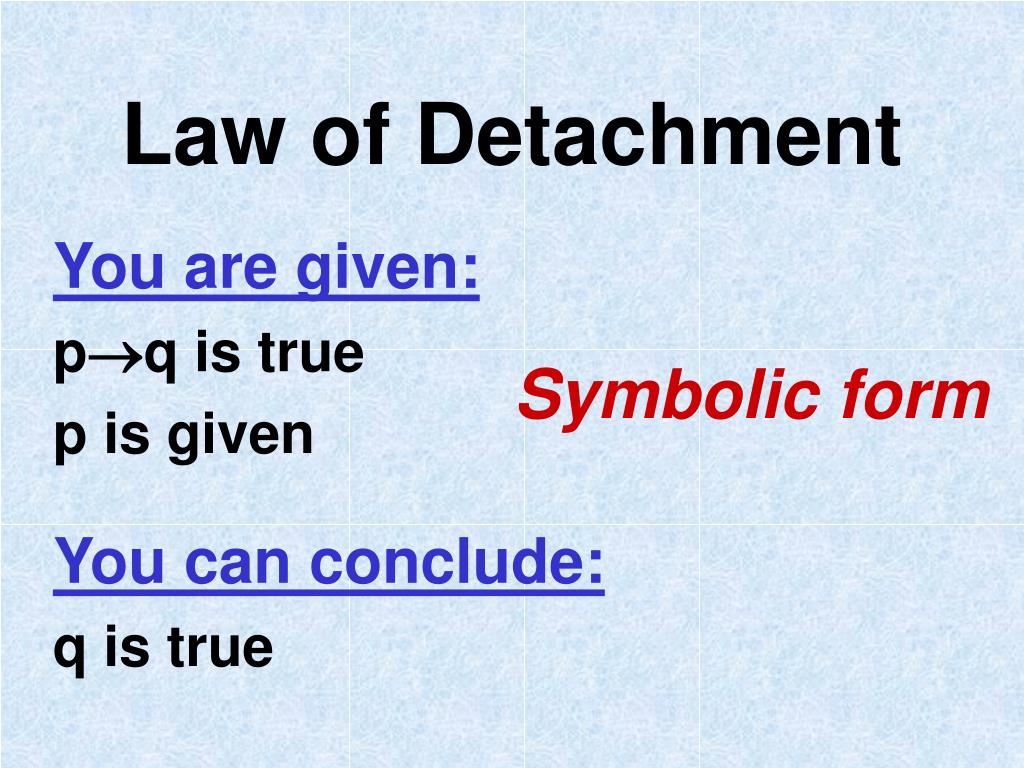
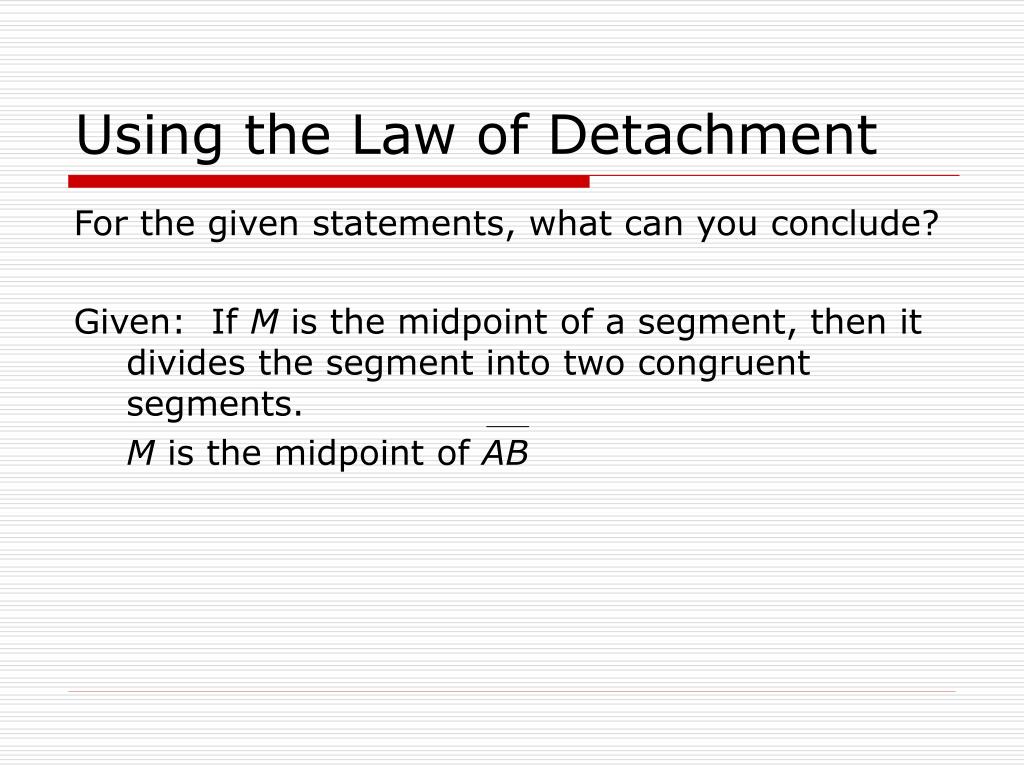
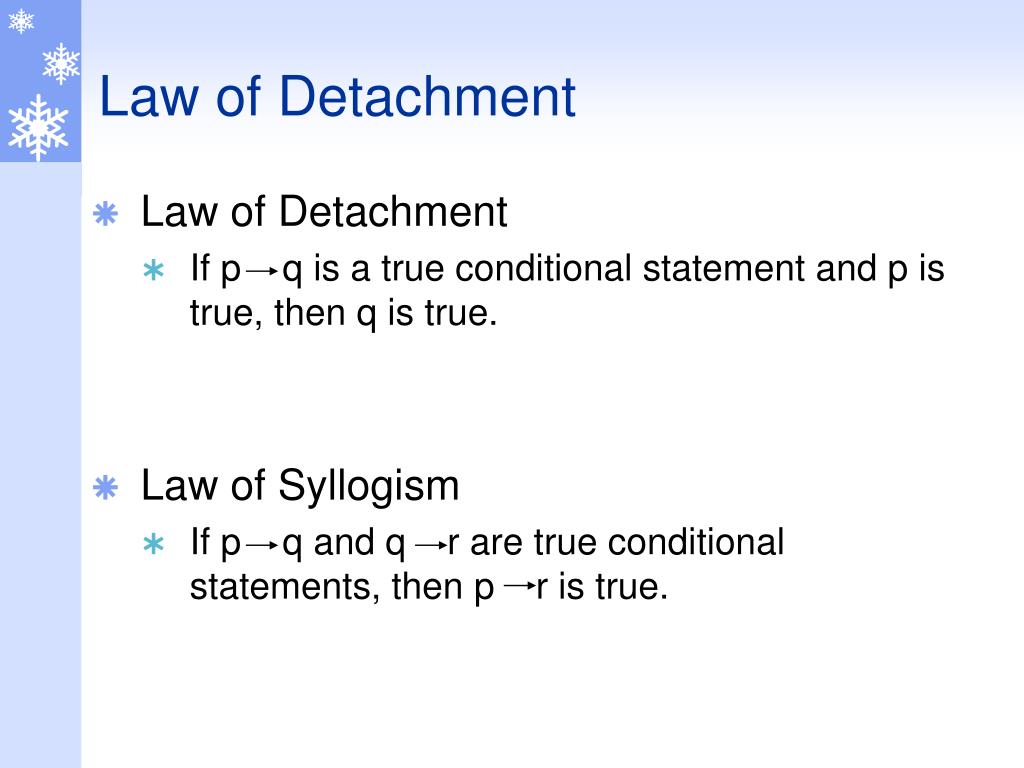
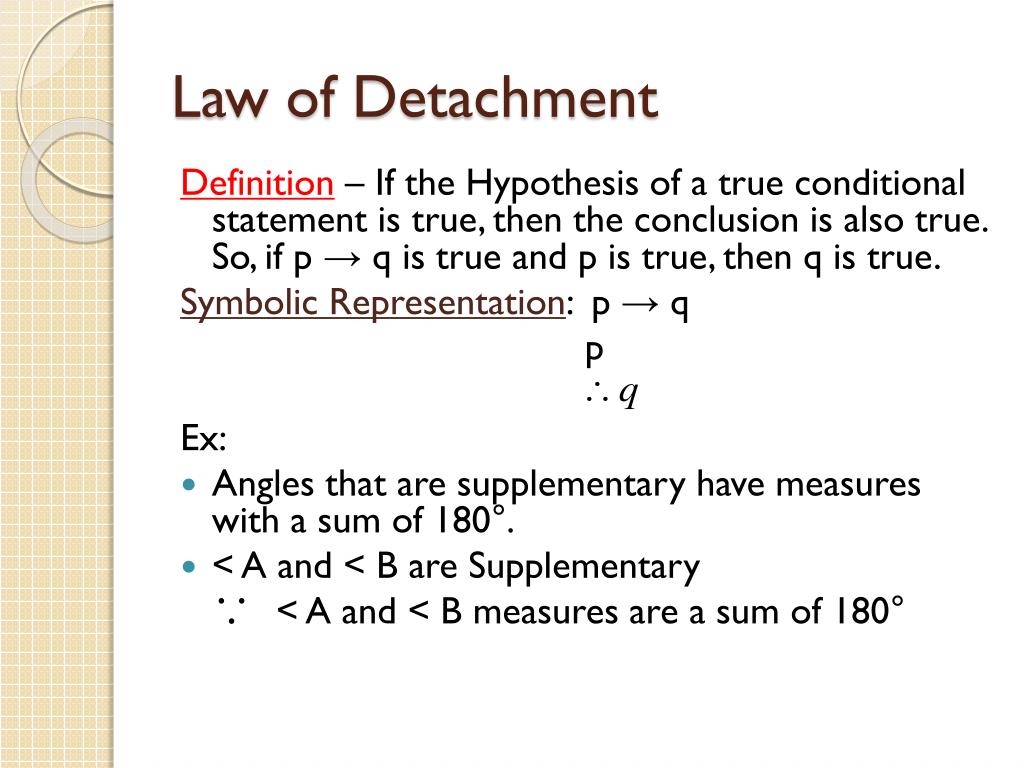
Law Of Detachment Symbolic Form
Law Of Detachment Symbolic Form - If p → q is true, and p is true, then q is true. P → q is a true statement since we all know that supposing. Web up to 6% cash back law of syllogism. Web law of detachment symbolic map. Web explore the law of detachment. Web in symbolic form: B → s b s premise: This argument has the structure described by the law of detachment. \(\begin{array} {ll} \text{premise:} & b \rightarrow s \\ \text{premise:} & b \\ \text{conclusion:} & s \end{array}\) this argument has the structure. Web up to 24% cash back a. (the second premise and the conclusion are simply the two parts of the. P → q is a true statement since we all know that supposing. Write as a conditional ex: This argument has the structure described by the law of detachment. (1) if p p , then q q. Find the definition of the law of detachment and understand its various scenarios. B → s b s premise: (1) if p p , then q q. Web up to 6% cash back law of syllogism. Web when using deductive reasoning there are a few laws that are helpful to know. Using r, s, v and the proper logic connectives, express the given premises in symbolic form. Web in symbolic form, if p → q is a true statement and p is true, then q is truth. If p s q is a true statement and p is true,. (the second premise and the conclusion are simply the two parts of. This argument has the structure described by the law of detachment. Web the law of detachment is a form of deductive reasoning that is used to draw conclusions. If mark saves $30, then he can buy a new video game. Web when using deductive reasoning there are a few laws that are helpful to know. If p → q is. Let use apply this to our example above. Web it introduces the law of detachment, law of syllogism, and law of contrapositive through statements about fictional wimborts, zeppies, and gloots. If mark saves $30, then he can buy a new video game. Web in symbolic form: P → q p ∴ q ∴ symbol for ``therefore''. Web in symbolic form, if p → q is a true statement and p is true, then q is truth. Let use apply this to our example above. Write the conclusion of the argument in symbolic form. What is the symbolic form for the law of syllogism? If mark saves $30, then he can buy a new video game. What is the symbolic form for the law of syllogism? Web it introduces the law of detachment, law of syllogism, and law of contrapositive through statements about fictional wimborts, zeppies, and gloots. \(\begin{array} {ll} \text{premise:} & b \rightarrow s \\ \text{premise:} & b \\ \text{conclusion:} & s \end{array}\) this argument has the structure. (the second premise and the conclusion are. Web explore the law of detachment. P → q p ∴ q ∴ symbol for ``therefore''. (2) p then we can derive a third true statement: Web what is the symbolic form for the law of detachment? Uses specific examples and patterns to form a. Web explore the law of detachment. Web the symbolic form is: If p s q is a true statement and p is true,. Web up to 6% cash back in mathematical logic, the law of detachment says that if the following two statements are true: P → q p ∴ q ∴ symbol for ``therefore''. Web up to 6% cash back in mathematical logic, the law of detachment says that if the following two statements are true: Web in symbolic form: Find the definition of the law of detachment and understand its various scenarios. B → s b s premise: Web explore the law of detachment. All deductive arguments that follow this pattern have a special name, the law of detachment. What is the symbolic form for. \(\begin{array} {ll} \text{premise:} & b \rightarrow s \\ \text{premise:} & b \\ \text{conclusion:} & s \end{array}\) this argument has the structure. Web explore the law of detachment. See examples of the law of detachment. Web the law of detachment applies when a conditional and its antecedent are given as premises, and the consequent is the conclusion. Web the law of detachment is a form of deductive reasoning that is used to draw conclusions. Web up to 24% cash back a. This argument has the structure described by the law of detachment. Web in symbolic form: (1) if p p , then q q. Web the symbolic form is: Uses specific examples and patterns to form a. If mark saves $30, then he can buy a new video game. Web up to 6% cash back law of syllogism. Let use apply this to our example above. P → q p ∴ q ∴ symbol for ``therefore''. If p → q is true, and p is true, then q is true. (2) p then we can derive a third true statement: What is the symbolic form for the law of syllogism?PPT Venn Diagrams and Logic PowerPoint Presentation, free download
Law of Detachment
PPT Venn Diagrams and Logic PowerPoint Presentation, free download
PPT 2.3 Deductive Reasoning PowerPoint Presentation, free download
PPT 2.3 Deductive Reasoning PowerPoint Presentation, free download
The Law of Detachment A Series on the Universal Laws
Law of Detachment Affirmations, Awakening quotes, Wisdom
PPT 2.1 Conditional Statements PowerPoint Presentation, free download
PPT Lesson 2.1 PowerPoint Presentation, free download ID3422718
PPT Deductive Reasoning PowerPoint Presentation, free download ID
Related Post: