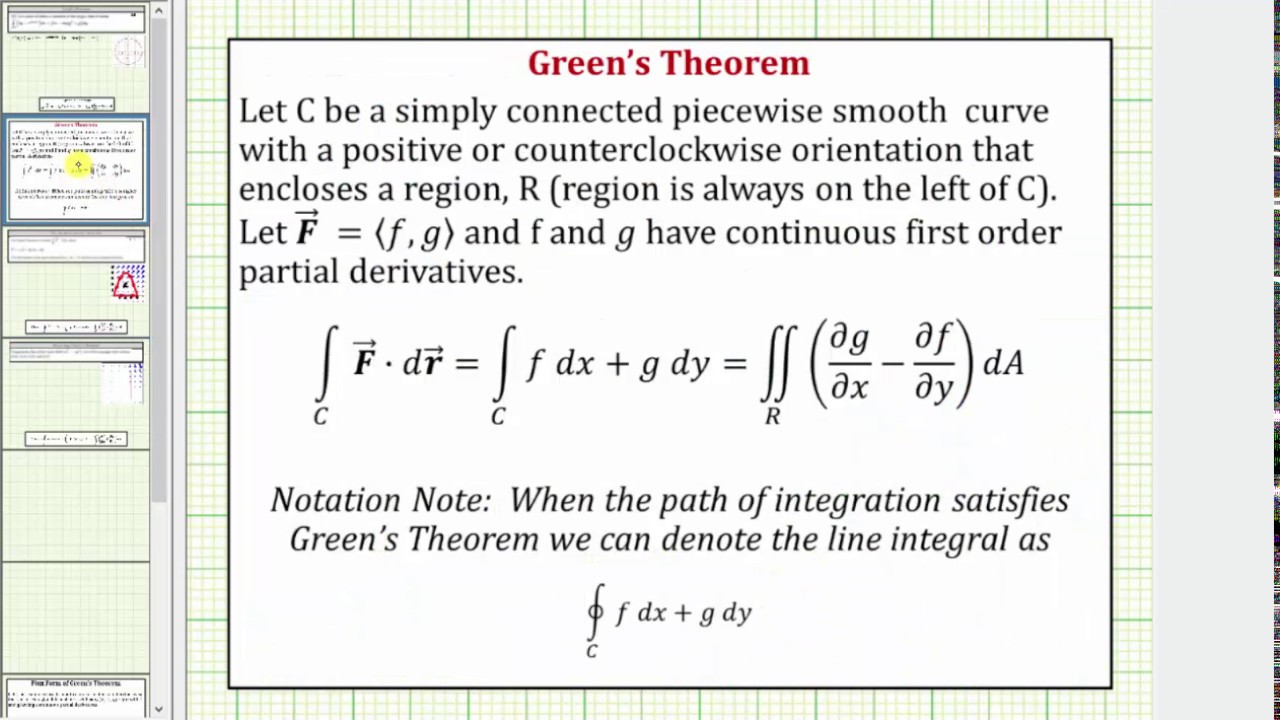
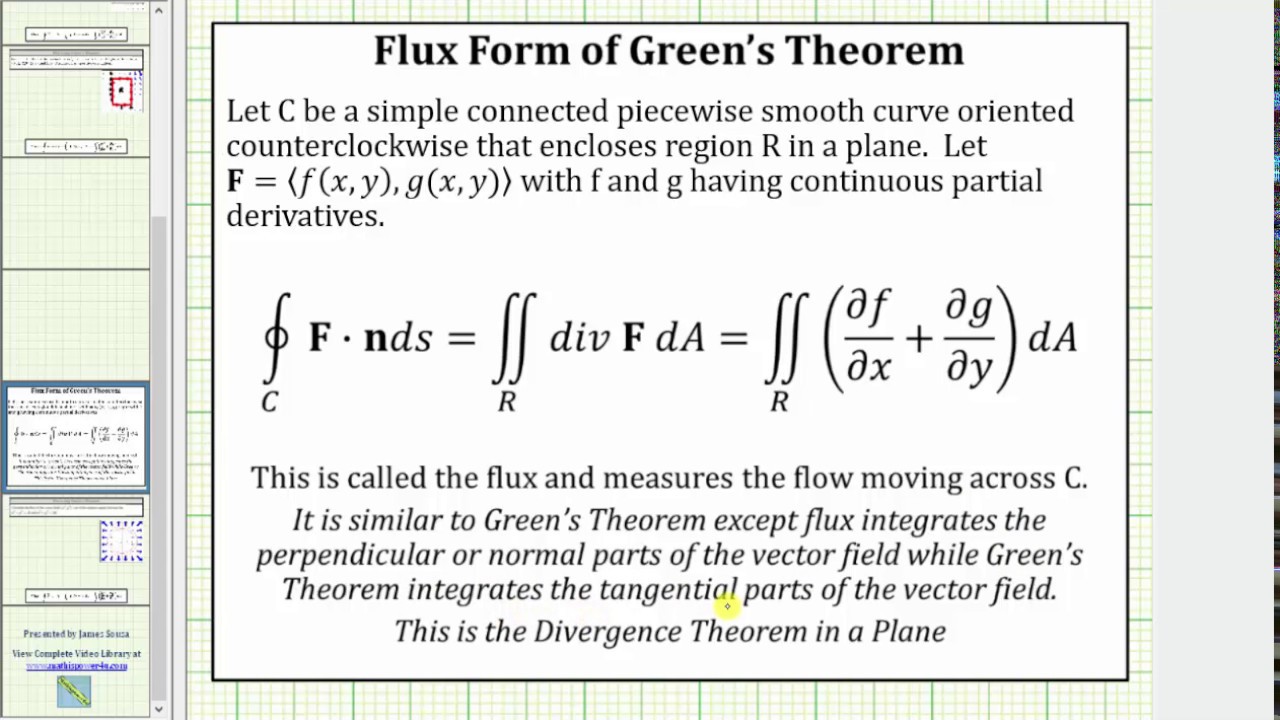
Green's Theorem Flux Form

Green's Theorem Flux Form - Circulation form) let r be a region in the plane with boundary curve c and f = (p,q) a vector field defined on r. Web circulation form of green's theorem. Web the flux form of green’s theorem relates a double integral over region d to the flux across boundary c. This theorem shows the relationship between a line. Web (1) flux of f across c = notice that since the normal vector points outwards, away from r, the flux is positive where the flow is out of r; Green’s theorem is mainly used for the integration of the line combined with a curved plane. Web introduction to flux form of green's theorem. Web all contents ©2019 arizona board of regents. We explain both the circulation and flux forms of. If p p and q q.

Determine the Flux of a 2D Vector Field Using Green's Theorem (Hole

Circulation form) let r be a region in the plane with boundary curve c and f = (p,q) a vector field defined on r. Web (1) flux of f across c = notice that since the normal vector points outwards, away from r, the flux is positive where the flow is out of r; Green's, stokes', and the divergence theorems..

Multivariable Calculus Green's Theorem YouTube

Green's theorem is a vector identity which is equivalent to the curl. Web introduction to flux form of green's theorem. Web (1) flux of f across c = notice that since the normal vector points outwards, away from r, the flux is positive where the flow is out of r; Let c c be a positively oriented, piecewise smooth, simple,.

Green's Theorem (Circulation & Flux Forms with Examples) YouTube

Then (2) z z r. Web green’s theorem is a version of the fundamental theorem of calculus in one higher dimension. Math > multivariable calculus > green's, stokes', and the divergence theorems > green's theorem. In the circulation form, the integrand is \vecs f·\vecs t. In the flux form, the.

[Solved] How are the two forms of Green's theorem are 9to5Science

Green's, stokes', and the divergence theorems. Web the flux form of green’s theorem relates a double integral over region d to the flux across boundary c. Flow into r counts as negative flux. © 2023 khan academy terms of use. Then (2) z z r.

Green's Theorem Flux Form YouTube

Green's theorem is a vector identity which is equivalent to the curl. ∮cp dx+qdy= ∬dqx −p yda ∮ c p d x + q d y = ∬ d q x − p y d a, where c c is the boundary of d d. We explain both the circulation and flux forms of. A circulation form and a flux.

Green's Theorem Flux Form YouTube

Web calculus and analysis. Web introduction to flux form of green's theorem. Flow into r counts as negative flux. Web (1) flux of f across c = notice that since the normal vector points outwards, away from r, the flux is positive where the flow is out of r; In vector calculus, green's theorem relates a line integral around a.

Flux Form of Green's Theorem YouTube

Flow into r counts as negative flux. Web (1) flux of f across c = notice that since the normal vector points outwards, away from r, the flux is positive where the flow is out of r; Web the flux form of green’s theorem relates a double integral over region d to the flux across boundary c. ∮cp dx+qdy= ∬dqx.

Determine the Flux of a 2D Vector Field Using Green's Theorem (Parabola

Flow into r counts as negative flux. Web all contents ©2019 arizona board of regents. Green's, stokes', and the divergence theorems. ∮cp dx+qdy= ∬dqx −p yda ∮ c p d x + q d y = ∬ d q x − p y d a, where c c is the boundary of d d. Web green’s theorem is a version.

Determine the Flux of a 2D Vector Field Using Green's Theorem

In the circulation form, the integrand is \vecs f·\vecs t. Flow into r counts as negative flux. Green's, stokes', and the divergence theorems. Web calculus and analysis. Web (1) flux of f across c = notice that since the normal vector points outwards, away from r, the flux is positive where the flow is out of r;

Flux Form of Green's Theorem Vector Calculus YouTube

Green’s theorem comes in two forms: This theorem shows the relationship between a line. If p p and q q. A circulation form and a flux form. In the circulation form, the integrand is \vecs f·\vecs t.

Web (1) flux of f across c = notice that since the normal vector points outwards, away from r, the flux is positive where the flow is out of r; Then (2) z z r. Green's, stokes', and the divergence theorems. Web circulation form of green's theorem. The flux of a fluid across a curve can be difficult to calculate using the flux. Web the flux form of green’s theorem relates a double integral over region d to the flux across boundary c. Web use the circulation form of green's theorem to rewrite ∮ c 4 x ln ( y) d x − 2 d y as a double integral. Green’s theorem comes in two forms: © 2023 khan academy terms of use. If p p and q q. Green's theorem is a vector identity which is equivalent to the curl. ∮cp dx+qdy= ∬dqx −p yda ∮ c p d x + q d y = ∬ d q x − p y d a, where c c is the boundary of d d. Web calculus and analysis. Web introduction to flux form of green's theorem. Circulation form) let r be a region in the plane with boundary curve c and f = (p,q) a vector field defined on r. Flow into r counts as negative flux. A circulation form and a flux form. Web green’s theorem is a version of the fundamental theorem of calculus in one higher dimension. Let c c be a positively oriented, piecewise smooth, simple, closed curve and let d d be the region enclosed by the curve. In vector calculus, green's theorem relates a line integral around a simple closed curve c to a double integral over the plane region d bounded by c.

Related Post:

• Tarrant County Service Request Form

---

• Proof Of Service Form California Family Law

---

• 1040 B Form

---

• Mcdonalds Job Application Form

---

• Form Vtr 60

---