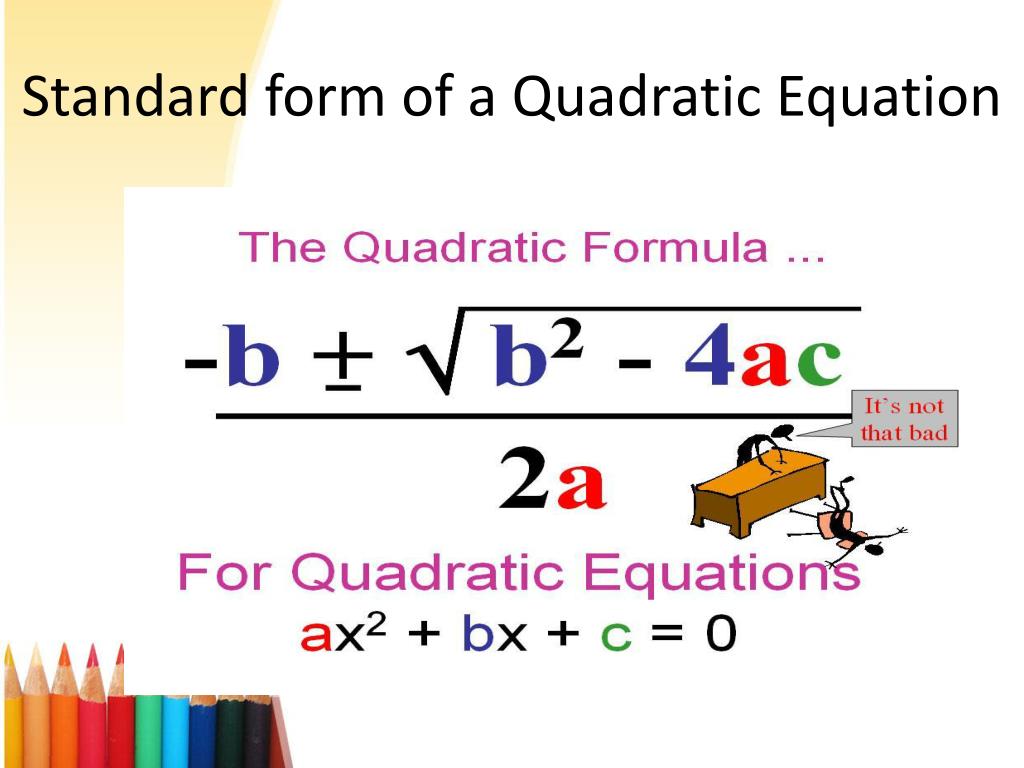
Derivative Quadratic Form

Derivative Quadratic Form - Web i would like to derive the derivative of the quadratic form $\frac{d}{dx} x^t ax$ using directional derivative. In the cases of one, two, and three variables they are called unary, binary, and ternary and have the. Quadratic formula (example 2) worked example: I want to compute the derivative w.r.t. $f(x) = x^tax$ is a function. Web a quadratic form involving n real variables x_1, x_2,., x_n associated with the n×n matrix a=a_(ij) is given by q(x_1,x_2,.,x_n)=a_(ij)x_ix_j, (1) where einstein. Web q(\twovecx1x2) = \twovecx1x2 ⋅ ([1 2 2 1]\twovecx1x2) = \twovecx1x2 ⋅ \twovecx1 + 2x22x1 + x2 = x2 1 + 2x1x2 + 2x1x2 + x2 2 = x2 1 + 4x1x2 + x2 2. Web so let us consider a function f(x): The quadratic form in variables x1, x2. N×n with the property that.

PPT 5. QUADRATIC EQUATIONS PowerPoint Presentation, free download

Web steps on how to derive the quadratic formula. Rd → rd f ( x): Web so let us consider a function f(x): Web derivation of quadratic formula. That formula looks like magic, but you can follow the steps.

Forms of a Quadratic Math Tutoring & Exercises

Quadratic formula (negative coefficients) quadratic. Algebra geometry trigonometry calculus number theory combinatorics probability We denote the identity matrix (i.e., a matrix with all. Web expressing a quadratic form with a matrix. Web derivation of quadratic formula.

260 [ENG] derivative of xT A x quadratic form YouTube

Y = 0 y = 0 in the general form of the quadratic function. Web quadratic forms are homogeneous quadratic polynomials in n variables. $f(x) = x^tax$ is a function. Notice that the derivative with respect to a. Web derivation of quadratic formula.

Standard Form of Quadratic Equation with Examples

“ xax, xy , for. Derivation of the quadratic formula is easy! Web i would like to derive the derivative of the quadratic form $\frac{d}{dx} x^t ax$ using directional derivative. Web i know that $a^hxa$ is a real scalar but derivative of $a^hxa$ with respect to $a$ is complex, $$\frac{\partial a^hxa}{\partial a}=xa^*$$ why is the derivative. Quadratic formula (example 2).

The derivative of a quadratic function YouTube

Web q(\twovecx1x2) = \twovecx1x2 ⋅ ([1 2 2 1]\twovecx1x2) = \twovecx1x2 ⋅ \twovecx1 + 2x22x1 + x2 = x2 1 + 2x1x2 + 2x1x2 + x2 2 = x2 1 + 4x1x2 + x2 2. = q for all i, j = 1,. Web $\begingroup$ the derivative of a function $f:\bbb{r^n}\to\bbb{r^m}$ is always an $m\times n$ linear map (matrix). Web.

The Quadratic Formula INTUITIVE Derivation Quadratics, Quadratic

Is there a way to calculate the derivative of a quadratic form. How to write an expression like ax^2 + bxy + cy^2 using matrices and vectors. A quadratic equation looks like this: Single variable case via quadratic approximation. A “ « a b{2 b{2 c.

Derivation of Quadratic Formula Solution Of Quadratic Equation YouTube

Web 7 quadratic forms 13 8 partitioned matrices 15 9 derivatives with matrix algebra 16 10 kronecker product 18 references 19 formula sources and proofs 20 version: Web i would like to derive the derivative of the quadratic form $\frac{d}{dx} x^t ax$ using directional derivative. Web the rule for when a quadratic form is always positive or always negative translates.

How to derive the Quadratic Formula YouTube

A quadratic equation looks like this: = q for all i, j = 1,. Quadratic formula (example 2) worked example: The quadratic form in variables x1, x2. Ad probability using permutations and combinations.

Quadratic Equation Derivation Quadratic Equation

In the n variables found in x. ∂xtax ∂x =xt(a +at) ∂ x t a x ∂ x = x t ( a + a t) using the chain rule of matrix differentiation?. Y = 0 y = 0 in the general form of the quadratic function. = q for all i, j = 1,. $f(x) = x^tax$ is a.

Derivation of the Quadratic Formula YouTube

Web steps on how to derive the quadratic formula. We can alternatively define a matrix q to be symmetric if. “ xax, xy , for. Then expanding q(x + h) − q(x) and dropping the higher order term, we get dq(x)(h) = xtah + htax = xtah + xtath = xt(a + at)h, or more typically, ∂q ( x) ∂x.

Derivation of the quadratic formula is easy! ∂xtax ∂x =xt(a +at) ∂ x t a x ∂ x = x t ( a + a t) using the chain rule of matrix differentiation?. R d → r d. N×n with the property that. I have saw different derivations (for example, this one, which. Is there a way to calculate the derivative of a quadratic form. $f(x) = x^tax$ is a function. Ad probability using permutations and combinations. A quadratic equation looks like this: Quadratic formula (example 2) worked example: Web $\begingroup$ the derivative of a function $f:\bbb{r^n}\to\bbb{r^m}$ is always an $m\times n$ linear map (matrix). I want to compute the derivative w.r.t. Differential forms provide an approach to multivariable calculus that is independent of coordinates. Lecture 15 symmetric matrices, quadratic forms, matrix norm, and svd. Single variable case via quadratic approximation. We can alternatively define a matrix q to be symmetric if. Web so let us consider a function f(x): X ∈ rd x ∈ r d of an expression that contains a quadratic. In the cases of one, two, and three variables they are called unary, binary, and ternary and have the. “ xax, xy , for.

Related Post:

• How To Fill Out A Renunciation Form Pa

---

• Virgina State Tax Form

---

• Ihss Tax Exempt Form

---

• Cooler 5th Form

---

• How Does A Convex Mirror Form An Image

---