Circulation Form Of Green's Theorem
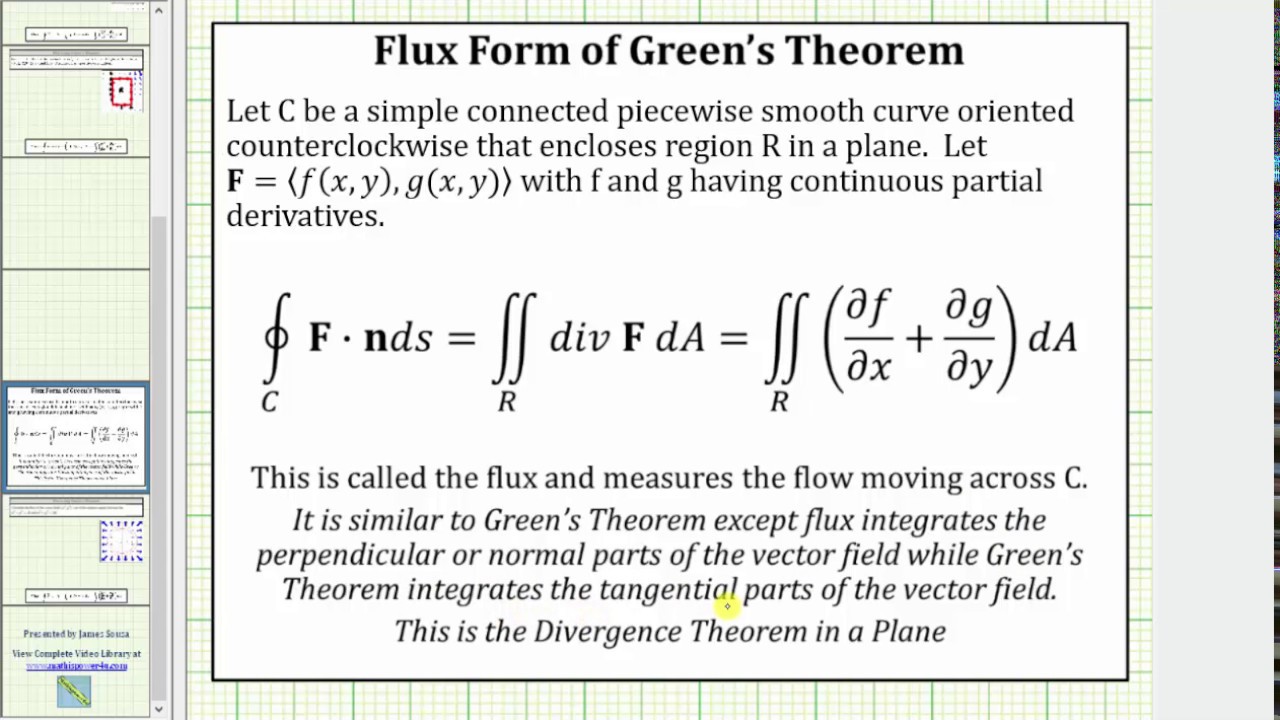
Circulation Form Of Green's Theorem - This form of the theorem relates the vector line integral over a simple, closed plane curve. Math > multivariable calculus > green's, stokes', and the divergence. Web determine the flux of a 2d vector field using green's theorem. A circulation form and a flux form, both of which require region d in the double integral to be simply connected. Web green’s theorem is a version of the fundamental theorem of calculus in one higher dimension. However, we will extend green’s. Let r be the region enclosed by c. Web circulation form of green's theorem math > multivariable calculus > green's, stokes', and the divergence theorems > green's theorem © 2023 khan academy terms of use. Use the circulation form of green's theorem to rewrite ∮ c 4 x ln ( y) d x − 2 d y as a double integral. If p p and q q. This form of the theorem relates the vector line integral over a simple, closed plane curve. A circulation form and a flux. Web green’s theorem is a version of the fundamental theorem of calculus in one higher dimension. Web circulation form of green's theorem. Web green's theorem, circulation form In the circulation form, the integrand is \vecs f·\vecs t. However, we will extend green’s. Web circulation form of green's theorem. Web green’s theorem let c c be a positively oriented, piecewise smooth, simple, closed curve and let d d be the region enclosed by the curve. Trefor bazett 287k subscribers 70k views 2 years ago calculus iv: Green’s theorem comes in two forms: Web circulation form of green's theorem math > multivariable calculus > green's, stokes', and the divergence theorems > green's theorem © 2023 khan academy terms of use. Web use the circulation form of green's theorem to calculate ∮ c f ⋅ d r where f (x, y) = 2 (x 2 + y 2),. Let r be the region enclosed by c. Web the first form of green’s theorem that we examine is the circulation form. Web green’s theorem has two forms: A circulation form and a flux form. Web green's theorem, circulation form Web circulation form of green's theorem. Web green’s theorem is a version of the fundamental theorem of calculus in one higher dimension. Web the two forms of green’s theorem green’s theorem is another higher dimensional analogue of the fundamental theorem of calculus: Web theorem 1.1 (green’s theorem for circulation). A circulation form and a flux form, both of which require. Web 0:00 / 7:54 curl vs circulation curl, circulation, and green's theorem // vector calculus dr. Web green’s theorem has two forms: Assume that c is a positively oriented, piecewise smooth, simple, closed curve. Web theorem 1.1 (green’s theorem for circulation). A circulation form and a flux form, both of which require region d in the double integral to be. A circulation form and a flux form, both of which require region d in the double integral to be simply connected. This form of the theorem relates the vector line integral over a simple, closed plane curve. Web circumference of a circle. Web the two forms of green’s theorem green’s theorem is another higher dimensional analogue of the fundamental theorem. Web theorem let c be a positively oriented, piecewise smooth, simple closed curve in a plane, and let d be the region bounded by c. Use the circulation form of green's theorem to rewrite ∮ c 4 x ln ( y) d x − 2 d y as a double integral. Circulation form of green's theorem. Green’s theorem shows the. Web green’s theorem let c c be a positively oriented, piecewise smooth, simple, closed curve and let d d be the region enclosed by the curve. Web theorem 1.1 (green’s theorem for circulation). Visit byju’s to learn statement, proof, area,. Web circulation form of green's theorem. Web green’s theorem has two forms: Green’s theorem comes in two forms: Web use the circulation form of green's theorem to calculate ∮ c f ⋅ d r where f (x, y) = 2 (x 2 + y 2), x 2 + y 2 , and c follows the graph of y = x 3 from (1, 1) → (3, 27) and then. Web circumference of. A circulation form and a flux form, both of which require region d in the double integral to be simply connected. Use the circulation form of green's theorem to rewrite ∮ c 4 x ln ( y) d x − 2 d y as a double integral. Web use the circulation form of green's theorem to calculate ∮ c f ⋅ d r where f (x, y) = 2 (x 2 + y 2), x 2 + y 2 , and c follows the graph of y = x 3 from (1, 1) → (3, 27) and then. In the flux form, the. Web circulation form of green's theorem math > multivariable calculus > green's, stokes', and the divergence theorems > green's theorem © 2023 khan academy terms of use. Web determine the flux of a 2d vector field using green's theorem. Web 0:00 / 7:54 curl vs circulation curl, circulation, and green's theorem // vector calculus dr. A circulation form and a flux. If l and m are functions of (x, y) defined on an. Math > multivariable calculus > green's, stokes', and the divergence. Assume that c is a positively oriented, piecewise smooth, simple, closed curve. It relates the line integral of a vector. Web circumference of a circle. Web green’s theorem is a version of the fundamental theorem of calculus in one higher dimension. In the circulation form, the integrand is \vecs f·\vecs t. Web green’s theorem let c c be a positively oriented, piecewise smooth, simple, closed curve and let d d be the region enclosed by the curve. Let r be a region in r2, and let c be the boundary of r, oriented counterclockwise. Web the two forms of green’s theorem green’s theorem is another higher dimensional analogue of the fundamental theorem of calculus: Let f be a vector field. Green’s theorem comes in two forms:Flux Form of Green's Theorem YouTube
Curl, Circulation, and Green's Theorem // Vector Calculus YouTube
PPT Chapter 16 Vector Calculus PowerPoint Presentation, free
Determine the Flux of a 2D Vector Field Using Green's Theorem
Multivariable Calculus Green's Theorem YouTube
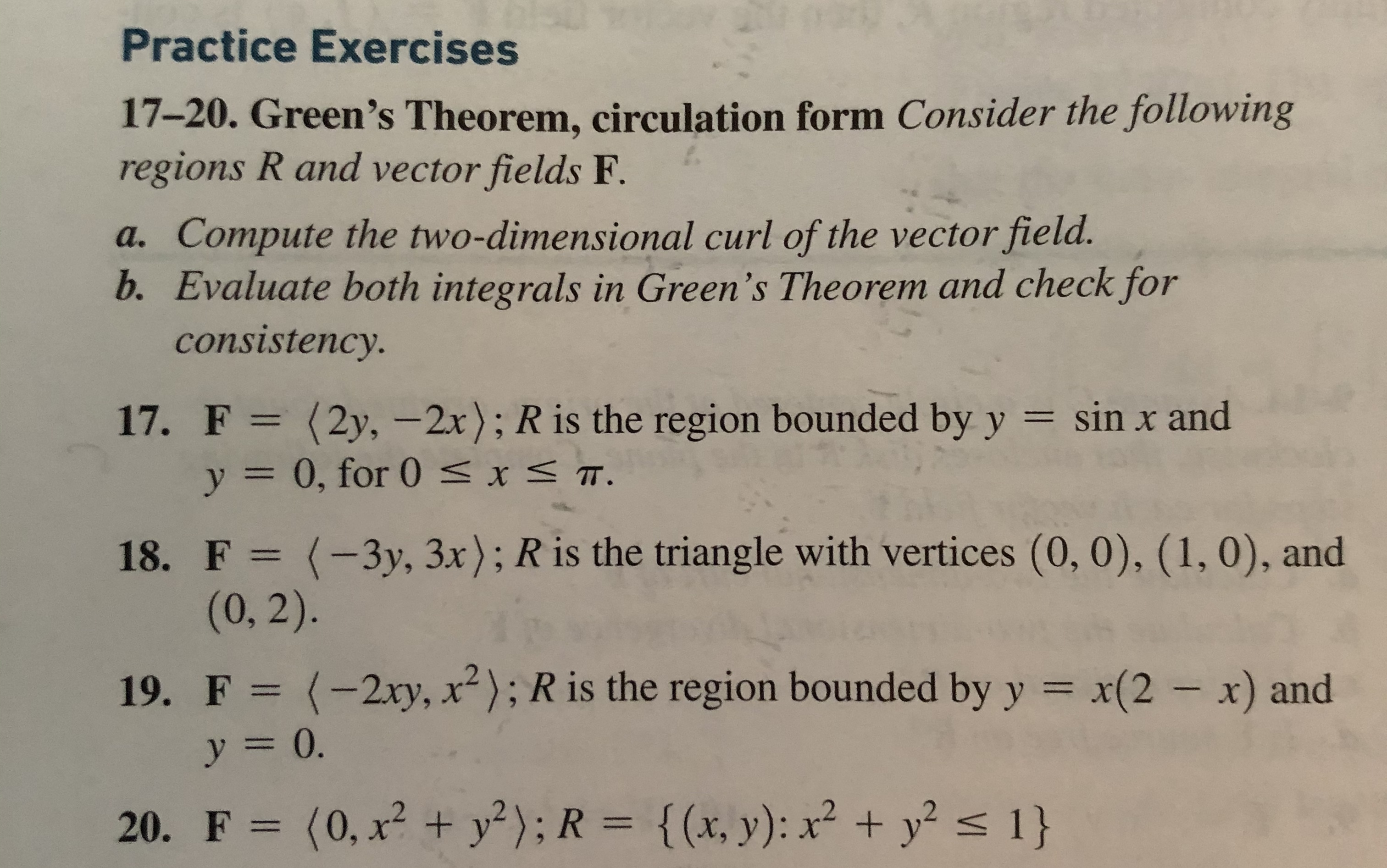
Answered 1720. Green's Theorem, circulation… bartleby
Green's Theorem, Circulation Form YouTube
Green's Theorem (Circulation & Flux Forms with Examples) YouTube
Application of Green's Theorem on upper half of the semi circle
[Math] How are the two forms of Green’s theorem are equivalent Math
Related Post:







![[Math] How are the two forms of Green’s theorem are equivalent Math](https://i.stack.imgur.com/ktYm1.png)